Why Mean, Median, and Mode Matter in Business
In the business world, data rules the game. Whether you're analyzing sales figures, customer demographics, or market trends, understanding mean, median, and mode can be your secret weapon. For instance, a retailer might use the median income of its target audience to set pricing strategies. Meanwhile, the mode could reveal the most popular product category. These tools help businesses make smarter decisions, ensuring they stay ahead of the competition.
Breaking Down the Empirical Relationship
Now, let's get into the nitty-gritty of how mean, median, and mode relate to each other. Picture this: a frequency distribution graph where the data points tell a story. The relationship can be divided into four cases, depending on the data's skewness. One key formula to remember is that the difference between thrice the median and twice the mean gives you the mode. It sounds complicated, but trust me, it's like solving a puzzle that makes sense when you break it down.
What Is the "Empirical Relationship"?
Here's a fun fact: this relationship between mean, median, and mode is called the "empirical relationship." In moderately skewed distributions, the mode equals the difference between three times the median and two times the mean. This formula might sound like a mouthful, but it’s a powerful tool statisticians use to decode data. Let's explore this concept in more detail to see how it all fits together.
Read also:Lee Mack The Comedy Legend You Cant Help But Love
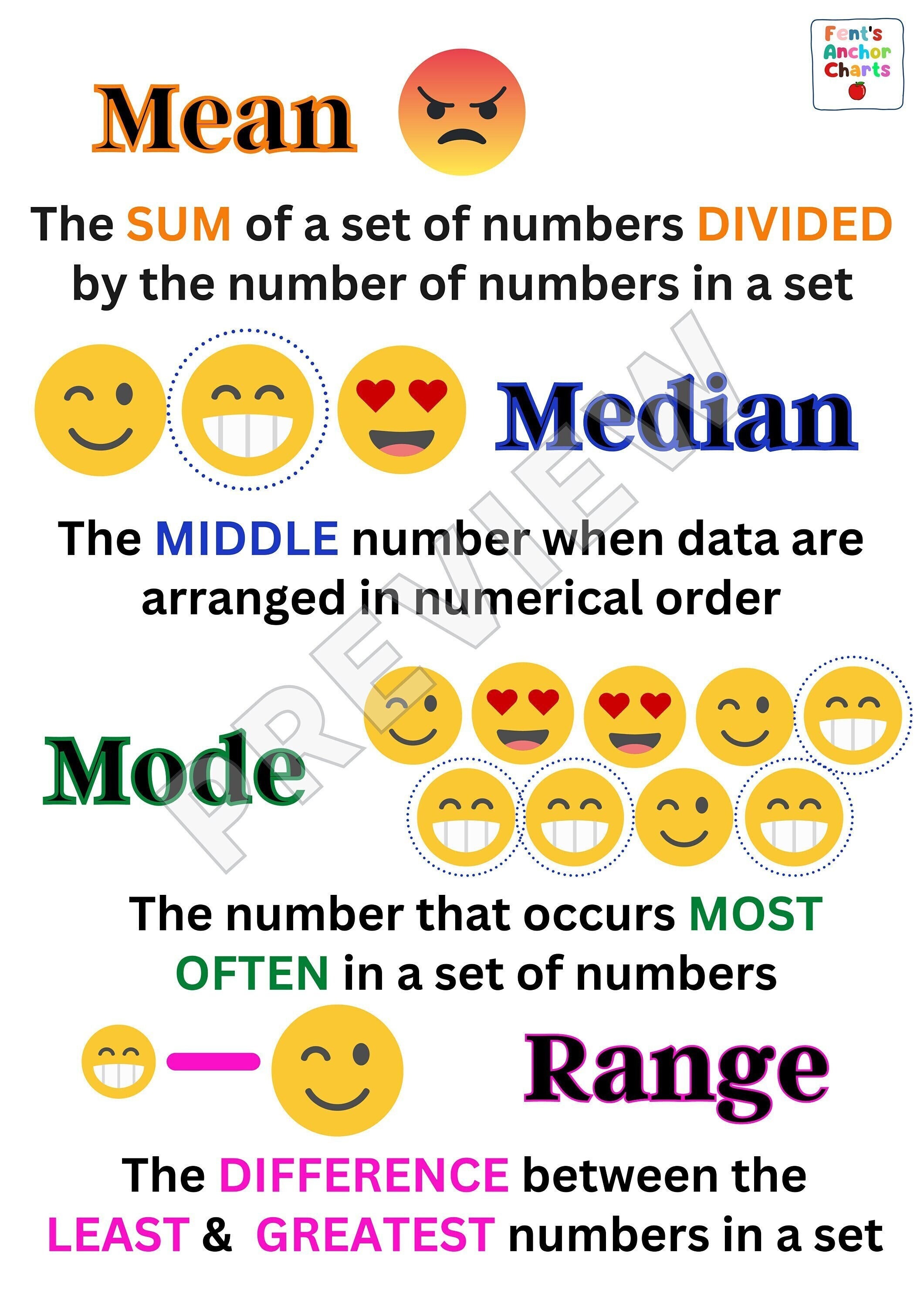
Central Tendency: The Backbone of Statistics
In statistics, we use three principal measurements to describe central tendency: the arithmetic mean, median, and mode. The arithmetic mean is like the "average Joe" of data—it takes all the values and condenses them into one number. The median is the middle value when the data is arranged in order, and the mode is the number that shows up the most. These tools are essential for simplifying large datasets into manageable insights.
Perfect Symmetry vs. Skewness
Imagine a perfectly symmetrical distribution where the mean and median are exactly the same. In this ideal scenario, the mode would also align with them. However, in the real world, data isn’t always so neat. When the data is skewed, the mean, median, and mode can differ significantly. This is where understanding their relationships becomes crucial for accurate analysis.
Practical Example: Solving for the Mean
Let's say you're given a list of numbers where the mode is 17 and the median is 29. Your task is to find the mean. Using the empirical formula, you can calculate that the mean is approximately 25. See how these values interconnect? It’s like a statistical dance where each step depends on the others.
When to Use Mean, Median, or Mode
Choosing the right measure of central tendency depends on the situation. For example, if your data has extreme values (outliers), the mean might not give you an accurate picture. In such cases, the median or mode could be better options. Think of it this way: if you're analyzing salaries in a company, a few high earners could skew the mean. The median, on the other hand, gives you a more realistic view of what the "average" employee earns.
Applications in Real Life
These statistical tools aren’t just for academics—they’re everywhere in real life. In healthcare, insurance analysts use mean, median, and mode to determine the average age of clients or the most common health conditions. Retailers use them to identify the most popular products. Even in education, teachers use these measures to understand student performance trends. The possibilities are endless!
Visualizing the Data
One of the best ways to grasp these concepts is by visualizing the data. Imagine a classroom where students are given a set of numbers to analyze. They calculate the mean, find the median by ordering the numbers, and identify the mode by spotting the most frequent value. Tools like diagrams and graphs can make this process much easier and more engaging.
Read also:Pam Van Sant A Life Of Love Loss And Legacy
Wrapping Up
In the world of statistics, mean, median, and mode are more than just numbers—they’re powerful tools that help us make sense of the world. Whether you're a business owner, a healthcare analyst, or just someone curious about data, understanding their relationships can open up new insights. So the next time you’re faced with a dataset, remember the empirical relationship and let the numbers tell their story.